Why: Practice
of addition Facts
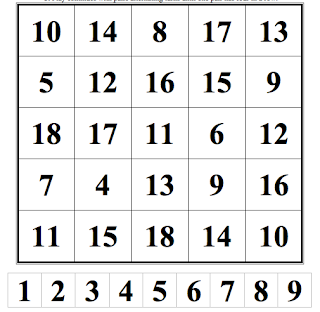
Object: To
cover four numbers in a row, horizontally, vertically, or diagonally
Students: Pair
players N.Z. Numeracy
Stage: Counting
on
Materials: Game
board, 2 sets of counters(two colours), 2 paper
clips
How: 1.
The first player places two paper clips on numbers at the bottom of the board,
adds them together and then places one of their counters on the sum on
the board
2.
The second pair moves JUST ONE paper clip to make a new sum and covers
the
appropriate numeral on the board.
3.
Play continues with pairs alternating turns until one pair has four in a row.
Adaptations: Change
numbers on the board and use three
1-6 die or 0-9 die
Adapted from Nimble With Numbers Grades 3/4
Note: 1. I prepare these type of activities on one side of an A4 or Quarto Card. This is so the rules and playing
board are on the same side, often I have seen games disrupted because the rules are on the reverse side of
the page!
2. I encourage students to play as a pair against another pair. With two in a 'team' there is a need for
maths discussion before a move is made. The more discussion the better the learning.